Primary pattern formation, organizing regions and regeneration
The problem of pattern formation
The generation of the complex structure of a higher organism within each life cycle is one of the most fascinating aspects of biology. The similarity of identical twins underlines of how reproducible this process can be when the genetic information is the same. Reference to the genes, however, does not provide on its own an explanation for the generation of spatial structures since with each cell division both daughter cells obtain, as the rule, the same genetic information. This leads to the question of how different parts of the developing organism can become different from each other. How can patterns emerge in an initially more or less structure-less system? How can different genetic information be activated at different positions?
Although many eggs have pronounced asymmetries, the final pattern cannot already be present in the egg in a hidden form. At early stages, many embryos can be fragmented into two parts and each part forms a complete organism. The sea urchin embryo at the 16-cell stage is an example. Thus, the structures must arise during development. Basic concepts for the underlying mechanisms have been derived from experiments involving perturbations of normal development.
We have used such observations to develop specific models for different developmental situations. By computer simulation we have shown that the regulatory features of the models correspond closely to the experimental observations. More recently, the new tools of molecular biology have opened the opportunity to clone genes and isolate molecules involved. In this way, several of these models have obtained direct support.
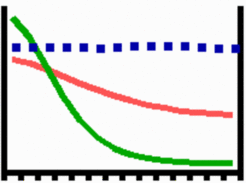
In his pioneering paper, Alan Turing [1] has shown in 1952 that pattern formation is possible by the interaction of two substances with different diffusion rates. We found that the crucial condition for pattern formation is local self-enhancement and long-range inhibition. A possible reaction consists of an activator (green) that has a strong feedback on its own productions rate. It controls also the production of its long ranging antagonist, the inhibitor. (In reality, more molecules could be involved to realize these positive and negative feedback loops.). A small elevation above a homogeneous steady state has a strong feedback on its further increase such that the deviation becomes more and more pronounced. The concomitantly produced inhibitor diffuses into the surroundings of this incipient maximum and regulates down the activation there. Random fluctuations in the ability to perform the reaction (blue squares) are sufficient for pattern initiation. Eventually, a stable pattern is formed in which the activator maximum is in balance with the surrounding cloud of inhibition. The antagonistic effect can also result from a depletion of a component that is required for the self-enhancing reaction (Gierer and Meinhardt, 1972 , [PDF] ; Gierer, 1981, [PDF] Meinhardt, 1982 [book-remake PDF]; 2003pattern7). Pattern formation is not a privilege of living systems. High sand dunes are formed although the sand is permanently redistributed by the blowing wind. Sharply contoured rivers are formed by erosion although the rain is more or less homogeneously distributed over a particular region. These and many other cases of inorganic pattern formation depend on the same principle.
Gradient formation
A pattern emerges whenever the size of the field becomes larger than the range of the activator. In fields with a size comparable to the activator range, the high activator concentration can be formed at one end of the field only. This is a very important aspect for the understanding of pattern formation in an early embryo: one side of the embryo becomes different from the other although the genetic information is the same in all cells. Activator maxima are assumed to act as organizing regions. The graded activator and/or inhibitor distribution can activate different genetic information in different parts of the tissue leading to an ordered sequence of structures in space (see Gene activation). Thus, the system is able to generate "positional information". In larger fields, several maxima and thus periodic structures can emerge.
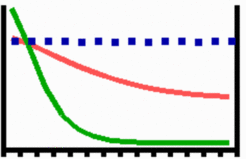
Pattern regeneration
An important feature of many developing systems is their ability to regenerate lost parts. For instance, in the freshwater polyp Hydra, after removal of a head a new head regenerates. Pattern regulation is a property of the activator-inhibitor system. As shown in the simulation above, with the removal of the activated region, also the inhibitor-producing cells are removed. The remnant inhibitor decays until a new maximum is formed via autocatalysis. Regeneration is only one of the most extreme manifestations of the self-regulation, an important component to make development error-tolerant and reproducible.
Example for an equation with pattern formation properties
In the models, equations are used that describe the concentration changes per time unit. These depend on the production, removal and exchange rates with neighboring cells (diffusion) and a small baseline (activator independent) production rate. The latter is required to initiate the reaction, for instance during regeneration or during oscillations. A typical equation for an interaction of an activator (a) and an inhibitor (h) is given below:
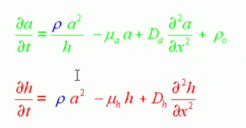
With such a set of equation, starting from certain initial concentrations, the concentration change in a short time interval can be calculated. Many such calculations allow computing the total time course. The animated simulations on this website are generated in this way.
The equation given is just one example for such pattern forming systems. We derived general criteria that allow us to design mechanisms, and assess equations as to their capabilities for pattern formation. For a short explication of 'A generative principle of pattern formation based on lateral inhibition, local instability and global stability' click here .
A short and simple BASIC program that can be run on any PC is given on a separate page . A detailed description of the properties of pattern forming reactions and software for the simulations on a PC is given elsewhere (Meinhardt, 2003).
Other molecular realizations
The activator-inhibitor mechanism is, of course, only an example, and other realizations are possible as well. The inhibition may not result from an inhibitor but from the depletion of a co-factor that is produced everywhere and which is consumed in the autocatalytic reaction. The autocatalysis can consist of a chain of reaction in which many molecules are involved. An example for an indirect autocatalysis is the mutual inhibition of two substances: the increase in the production of one substance leads to a reduction of the second, and thus to a further increase of the first, as if the first system would be autocatalytic.
The relation or our mechanism to that proposed by Turing [1] is described here.
The possible role of ß-catenin and Wnt in organizer formation of hydra is described here.
Further Reading and References
Gierer, A. and H. Meinhardt (1972), A theory of biological pattern formation. Kybernetik 12, 30-39. [PDF]
This paper introduces the concepts of autocatalysis and lateral inhibition underlying the generation of spatial concentration patterns. It derives mathematical criteria for non-linear interactions required for pattern generation, providing a method for designing molecular models. One of the simplest and most widely used example is bimolecular activation in conjunction with monomolecular inhibition. Striking features of developmental regulation can be accounted for along these lines.
Gierer, A. (1981) Generation of biological patterns and form: Some physical, mathematical, and logical aspects. Progr. Biophys. molec. Biol. 37, 1-47 [PDF].
The paper reviews models and mechanisms of biological pattern formation with emphasis on the roles of autocatalysis and lateral inhibition. In particular, it contains a demonstration that these two features are mathematically necessary for the simplest two-factor systems, and that they can be generalized, to a considerable extent, to apply to multicomponent systems of pattern formation.
Meinhardt, H. (1982) Models of biological pattern formation. (Academic Press London; a PDF - remake is freely available)
In the book, many models of biological pattern formation are discussed. Since it was definitely written before the molecular tools became available, the mechanisms proposed on the basis of modeling where clear predictions. Several mechanism central in biological pattern formation have been meanwhile confirmed.
- Turing, A. (1952). The chemical basis of morphogenesis. Phil. Trans. B. 237, 37-72. [available from the web]
[
[Next:
Feedback to: mailto:hans.meinhardt@tuebingen.mpg.de
| Meinhardt pages
Last update: April 26, 2006


