Formation of periodic structures
Periodic patterns are frequently formed in development. Examples are bristles, feather buds, leaves, and within the latter, stomata and trichoms. In the model, activator maxima are formed in a more or less regular arrangement if the size of the field is larger than the range of the inhibitor:

Insertion of new maxima during growth: It is a property of such a system that new peaks become inserted if due to growth the distances between existing maxima become too large. With increasing distance of the peeks the inhibitor becomes lower and lower until a new activation is triggered. In the course of time the new maximum becomes indistinguishable from the older maxima. Such an insertion of new pattern elements has been observed, for instance, by Wigglesworth in the bristles of a bug. After leach moult, new hairs appear at the largest hair-free regions. Likewise, in the blue-green alga Anabaena about every 7th cell is a heterocyst, a nitrogen-fixating cell [2]. During growth, new heterocysts are inserted whenever the distance of the existing heterocysts exceeds a certain limit. The molecular ingredients show self-enhancement (of a transcription factor) and long-range inhibition by a small peptide, in full agreement with our theory.
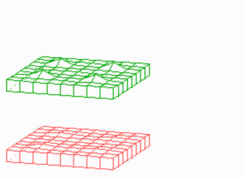
The periodic pattern becomes more regular if the pattern forming reaction works already during growth. Whenever the distance to an existing maximum becomes large enough, a new one is inserted. Below, a simple model for the initiation of leaf primordia on a growing shoot is given. Assumed is a cylinder that grows by cell proliferation at its upper end. Activator maxima are assumed to be the signal for leaf initiation. In this case, they appear during growth at alternating positions. (The existence of helical arrangements of leaves suggests a more complex mechanism, see Phyllotaxis )
Stripes are another periodic pattern that is frequently encountered in development. The zebra stripes are proverbial. Transitions between patch- and stripe-like patterns can be frequently seen on the skin pattern of tropical fishes. How can pattern elements emerge that have a long extension in one dimension but a narrow extension perpendicular to the first? According to the model, stripes can be formed if the rate of activator autocatalysis saturates at high activator concentrations. This leads also to a limitation of the inhibitor production. Since the activator production per cell is lower, more cells become activated until sufficient inhibitor is produced that abolish a further enlargement of the activated region. In other word, with saturation, the activated regions have the tendency to enlarge. However, in order to become activated, a close neighborhoodto non-activated cells is essential into which the inhibitor can be dumped. Both requirements, large activated patches and a direct nneighborhood of non-activated cells, seems to contradict each other. This is, however, not the case. In a stripe-like activation pattern, each activated cell has an activated nneighborand non-activated cells are close by. Kondo and Asai [7] observed regulating of striping patterns on tropical fishes and have shown that corresponding insertions and splitting can be explained using this type of model (for equation and parameters click here ).

Formation of activated cells in a certain proportion: In the mechanism described above, stripes will only appear if some activator diffusion takes place. This enforces that activated regions appear in more coherent regions. Without diffusion a salt-and-pepper pattern results in which the activated and non-activated cells have a certain proportion to each other. Such a mechanism is important, e.g., in the retina where a particular cell type has to be present in a particular proportion. The well-regulated ratio of pre-stalk and pre-spore cells is another example. In the model, activated cells can emerge at adjacent positions if the autocatalysis saturates (simulation below), without saturation the activated cells are separated due to lateral inhibition (first simulation on this page).

MOLECULES INVOLVED THE GENERATION OF PERIODIC STRUCTURES
In the Drosophila fly the precursor cells of the peripheral nervous system (neuroblasts) are derived from two lateral bands of ectodermal cells. Crucial for neuroblast formation are the genes of the Achaete-Scute complex. Both genes have a direct autocatalytic feedback on their own synthesis [1,6]. Cells that express these genes activate the gene Delta that codes for a molecule exposed at the cell surface. It stimulates the receptor Notch, a molecule ubiquitously present on all cells in that region and that acts ultimately on the Achaete-Scute complex in an inhibitory way. In this way, particular cells are singled out to participate in the formation of the peripheral nervous system while the remaining cells form the ectoderm.. Similar reactions have been found in the spacing of hairs on the leaves of Arabidopsis [3]. In the generation of highly regular feather patterns, BMP-2 and BMP-4 are involved in the lateral inhibition [5], while FGF-4 and sonic hedgehog are elements of the activating mechanism [4]. In these investigations, the regions in which genes for activating and inhibiting molecules are transcribed were determined. As expected from the theory, in most cases these regions coincide. A more direct comparison with the theory, however, would not only require a knowledge of regions where the molecules are produced but of the actual ranges of the signaling molecules.
Further Reading and References
Meinhardt, H. and Gierer, A. (1974). Applications of a theory of biological pattern formation based on lateral inhibition. J. Cell Sci. 15, 321-346. [PDF]
(see also remake of the book: Models of Biological Pattern Formation [PDF])
Stripe formation due to saturation of autocatalysis was first described in Meinhardt, 1989 [PDF].
More complex patterns require the superposition of several pattern forming systems, for instance to generate periodic elements that have an intrinsic polarity or to generate a strictly periodic pattern behind a wave that makes cells competent. Corresponding models can be found in:
Koch, A.J. and Meinhardt, H. (1994). Biological pattern formation - from basic mechanisms to complex structures. Rev. Modern Physics 66, 1481-1507. [PDF]
- Culi, J. and Modolell, J. (1998). Proneural gene self-stimulation in neural precursors - an essential mechanism for sense organ development that is regulated by notch signaling. Genes Dev. 12, 2036-2047.
- Golden, J.W. and Yoon, H.S. (2003): Heterocyst development in Anabaena. Curr. Opin.Microbiol 6,557-563
- Hülskamp,M. (2004). Plant trichomes: a model for cell differentiation Nat Rev Mol Cell Biol 5, 471-480
- Jung, H.S., Franciswest, P.H., Widelitz, R.B., Jiang, T.X., Tingberreth, S., Tickle, C., Wolpert, L. and Chuong, C.M. (1998). Local inhibitory-action of bmps and their relationships with activators in feather formation - implications for periodic patterning. Dev. Biol. 196, 11-23.
- Noramly, S. and Morgan, B.A. (1998). BMPs mediate lateral inhibition at successive stages in feather tract development. Development 125, 3775-3787.
- Van Doren, M., Powel, P.A., Pasternak, D., Singson, A. and Posakony, J.W. (1992). Spatial regulation of proneural gene activity: auto- and cross-achtivation of achaete is antagonized by extramacrochaetae. Genes Dev. 6, 2592-2605.
- Kondo, S. and Asai, R. (1995). A viable reaction-diffusion wave on the skin of Pomacanthus, the marine Angelfish. Nature 376, 765-768.




