Equations that describe of gene activation under the influence of morphogens

Simple threshold formation: The following equation allows a switch-like activation whenever the morphogen concentration m is above a certain threshold

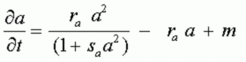
The gene activator a has a positive feedback on its own synthesis that saturates at high levels. It is cell-local, i.e., no diffusion takes place as appropriate for transcription factors; m is the concentration of the external signal, i.e., the local concentration of the morphogen gradient. With r_a = 0.05 (decay rate) and s_a = 0.2 (factor that determines the level of saturation) the switch occurs at about m = 0.08

At low levels of the gene activator and in the absence of any external signal m, the linear decay is dominating. The concentration will decrease further. In contrast, at higher levels of the signal the positive feedback becomes dominating. The concentration will increase until the saturation comes into play. Thus, there are only two stable states, low and high. If a cell is exposed to a sufficient morphogen concentration the autocatalysis is triggered and the low steady state may disappear. The concentration of the gene activator rises until the upper state is reached. The latter will be maintained even if the morphogen is no longer available: the cell changed its differentiation permanently. (This equation was first proposed 1976 for a model that accounts for the generation of net-like structures).

Unidirectional promotion: activation of several genes by a single gradient : The position-dependent activation of several genes by a single gradient can be achieved by the following type of interaction:

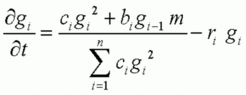
All genes involved are assumed to follow the same rules. Each gene product, g_i has an autocatalytic feedback on its own gene activation and an inhibitory action on the activation of alternative genes. This has the consequence that within a particular cell only one of the alternative genes can remain active. The self-inhibition of a gene has the character of a saturation and leads to an upper limit of the activation that a gene can obtain. The morphogen m has an additional activating influence on the genes. In this example it is assumed that the activation of the gene g_i+1 requires the presence of the gene product of the gene g_i. The terms c_i describe the ability of the genes to perform the auto regulation. For a position-dependent gene activation it is required that 'higher' genes are less sensitive to the morphogen but better in the mutual competition, i.e., the relation c_i+1 > c_i must be satisfied. The simulation shown was calculated with r_i = 0.01, c₁ = 0.01; c₂ = 0.01.42; c₃ = 0.02, etc.: m < 4.7 as plotted, b_i = 0.0019.

Equations that describe of gene activation under the influence of morphogens

Further Reading